jasonlarkin.github.io

Jason Larkin's MS Projects

Power-law distributions of particle concentration in free-surface flows

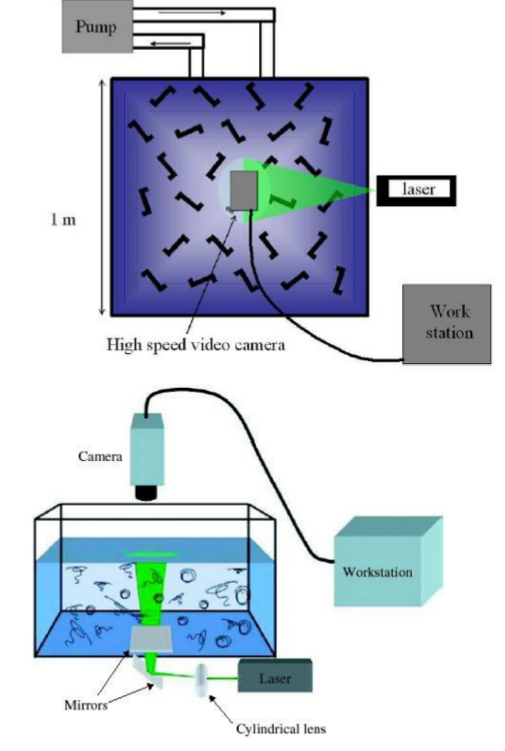
Building on the experiments performed for the project: Time-Evolution of a Fractal Distribution: Particle Concentrations in Free-Surface Turbulence we studied the statistics of particles floating on the surface of a turbulent fluid. The particles accumulate along string-like structures, while leaving large regions of the flow domain empty.

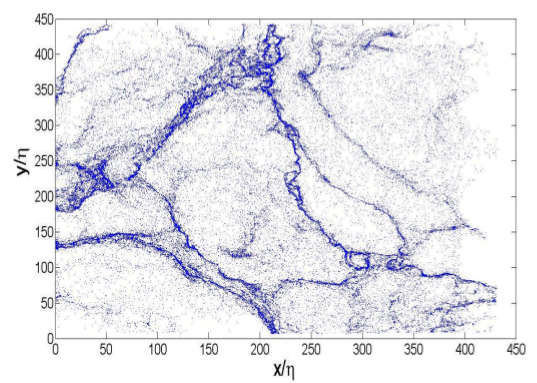
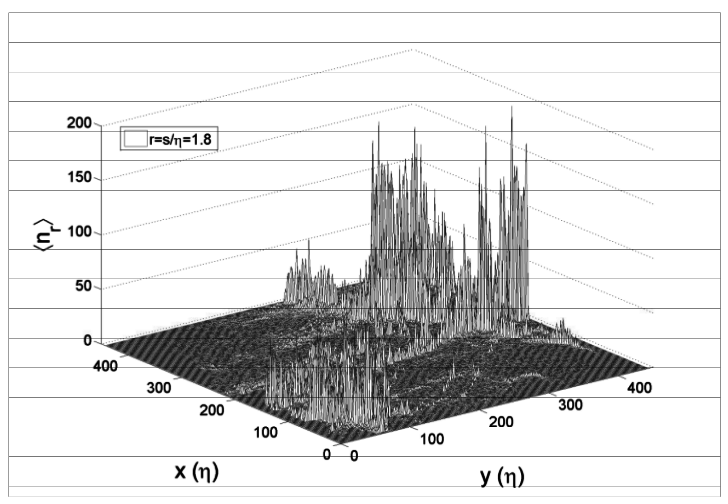
The steady-state particle distribution shows string-like coagulations surrounded by relatively depleted regions (left Figure). The concentration field, n_r, shows a very intermittent distribution in the steady state, with very large spikes concentrated along string-like structures, surrounded by vast depleted regions that are nearly flat (above Figures). Note that the parameter η is the Kolmogorov microscale, which is the smallest scale of the turbulent flow.

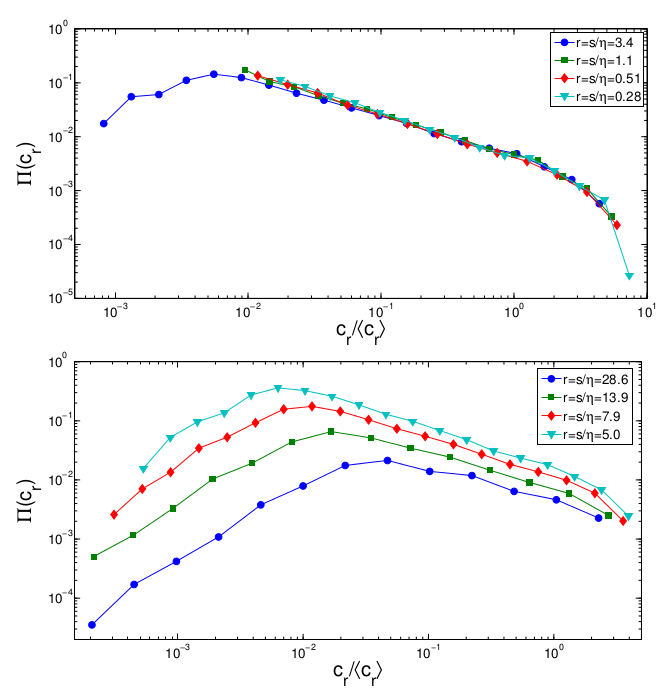
These regions of depleted concentration are reflected by a very peaked probability distribution function (PDF) of the Lagrangian concentration field c_r, Π(c_r), around c_r=0 (above Figures). The Lagrangian concentration field c_r is related to the Eulerian concentration field n_r through a simple transformation. The PDFs exhibit power-law behavior at small values of c_r for both the inertial range (r>1) and the dissipative range (r<1) of the flow, characterizing the very high probability of having regions containing very low concentrations. Understanding this particle clustering is of fundamental interest and practical use in a range of surface transport phenomena, such as the dispersion of phytoplankton or contaminants in oceanic flow.